Rounding decimals

When rounding decimals, you should be especially careful, because a decimal consists of a whole part and a fractional part. And each of these two parts has its ownplace values:

Whole place value:

• ones;
• tens;
• hundreds;
• thousands.

Fractional Part place value:

• tenths;
• hundredths;
• thousandths

Consider the decimal 123.456 - one hundred and twenty-three whole four hundred and fifty-six thousandths. Here the whole part is 123 and the fractional part is 456. Each of these parts has its own digits.

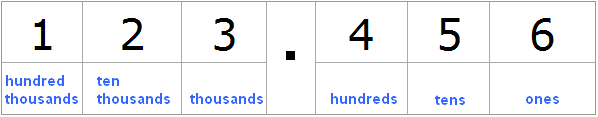
The same rounding rules apply to the whole part as to regular numbers. The difference is that after rounding the whole part and replacing all digits after the stored digit with zeros, the fractional part is completely discarded.

For example, round the decimal 123.456 to the place of tenth. It is very important not to mix up these digits. The digit of tens is in the whole part, and the digit of tens in the fractional part.

So, we have to round 123.456 to the place of tens. The stored digit here is 2, and the first of the discarded digits is 3

According to the rule, if the number you are rounding is followed by 0, 1, 2, 3 or 4, then the digit to be retained remains unchanged.

So the stored digit will remain unchanged and everything else will be replaced by zero. What about the fractional part? It is simply discarded (removed):

123.456 ≈ 120

Now let's try to round the same decimal 123.456 to ones. The retained digit here will be 3, and the first of the discarded digits is 4, which is in the fractional part:

According to the rule, if the first digit to be discarded is 0, 1, 2, 3 or 4 when rounding a number, then the digit to be retained remains unchanged.

So the retained digit will remain unchanged and everything else will be replaced by zero. The remaining fractional part will be discarded:

123.456 ≈ 123.0

The zero left after the decimal point can also be discarded. So the final answer will look like this:

123.456 ≈ 123.0 ≈ 123

Now let's deal with rounding of decimals. The same rules are valid for rounding fractions as for rounding integers. Let's try to round the fraction 123.456 to the decimal place. In the place of tenths there is digit 4, so it is a retained digit, and the first digit to be discarded is 5, which is in the place of hundredths:

According to the rule, if when rounding numbers, the first of the discarded digits is 5, 6, 7, 8 or 9, then the stored digit is incremented by one.

So the stored digit 4 will be incremented by one and the rest will be replaced by zeros

123.456 ≈ 123.500

Let us try to round the same fraction 123.456 to the hundredth place. The retained digit here is 5, and the first of the discarded digits is 6, which is in the thousandths digit:

According to the rule, if when rounding numbers, the first of the discarded digits is 5, 6, 7, 8 or 9, then the stored digit is incremented by one.

So the retained digit 5 will be incremented by one and the rest will be replaced by zeros

123.456 ≈ 123.460

Video lesson

Add comment