We continue to study elementary math tasks. This lesson is about motion tasks.
Task on finding the distance, speed, time
Task 1. A car moves at a speed of 80 km/h. How many kilometers will it travel in 3 hours?
Solution
If a car travels 80 kilometers in one hour, it will travel three times as much in three hours. To find the distance, multiply the speed of the car (80 km/h) by the travel time (3h)
80 × 3 = 240 km
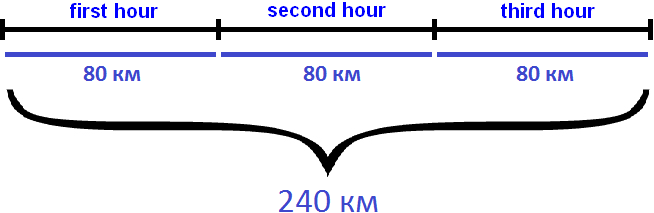
Answer: the car will drive 240 kilometers in three hours.
Task 2. The car drove 180 km with the same speed in 3 hours. Calculate the car's speed.
Solution
Speed is the distance traveled by a body in a unit of time. A unit is 1 hour, 1 minute, or 1 second.
If the car traveled 180 kilometers in 3 hours at the same speed, then by dividing 180 kilometers by 3 hours we will determine the distance the car traveled in one hour. And this is the speed of travel. To determine the speed, we need to divide the distance traveled by the time of travel:
180 : 3 = 60 km/h

Answer: the speed of the car is 60 km/h
Task 3. In 2 hours the car drove 96 km, and the cyclist drove 72 km in 6 hours. How many times faster did the car travel than the cyclist?
Solution
Determine the speed of the car. To do this, divide the distance traveled (96 km) by the time of its movement (2h)
96 : 2 = 48 km/h
Determine the speed of the cyclist. To do this, divide the distance traveled (72 km) by the time of his movement (6h).
72 : 6 = 12 km/h.
Find out how many times faster the car traveled than the cyclist. To do this, find the ratio of 48 to 12
![]()
Answer: the car was 4 times faster than the cyclist.
Task 4. A helicopter flew a distance of 600 km at a speed of 120 km/h. How long was it in flight?
Solution
If the helicopter can fly 120 kilometers in 1 hour, then we need to find out how many hours did helicopter spend flying this 600 kilometers. To find the time, divide the distance traveled by the speed of travel
600 : 120 = 5 hours
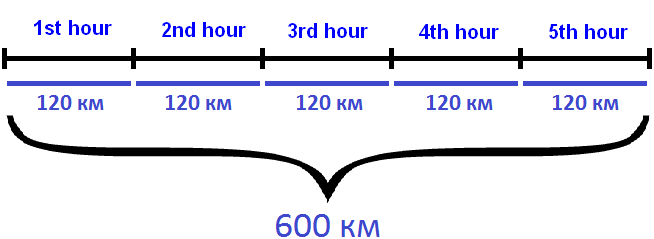
Answer: The helicopter was flying for 5 hours.
Task 5. A helicopter flew for 6 hours at a speed of 160 km/h. What distance did it travel during this time?
Solution
If the helicopter traveled 160 km in 1 hour, then it traveled six times more during 6 hour trip. To determine the distance, multiply the speed by the time
160 × 6 = 960 km

Answer: the helicopter covered 960 km in 6 hours.
Task 6. The car drove from Perm to Kazan (723 km) in 13 hours. For the first 9 hours it was driven at a speed of 55 km/h. Determine the speed of the car in the remaining time.
Solution
Determine how many kilometers the car traveled during the first 9 hours. For this purpose let's multiply the speed at which it drove during the first 9 hours (55 km/h) by 9
55 × 9 = 495 km
Let us determine the distance left to drive. To do this, subtract from the total distance (723 km) the distance he drove in the first nine hours
723 - 495 = 228 km
The car traveled the 228 km in the remaining 4 hours. To determine the speed of the car in the remaining time, divide 228 kilometers by 4 hours:
228 : 4 = 57 km/h
Answer: the speed of the car in the remaining time was 57 km/h
Two cars (vehicles) - math problem
For example, if two pedestrians start walking from two points to meet each other, and the speed of the first is 100 meters/min, and the second pedestrian is 105 meters/min, then both of them cover 205 meters/min. This means that every minute the distance between the pedestrians will decrease by 205 meters.
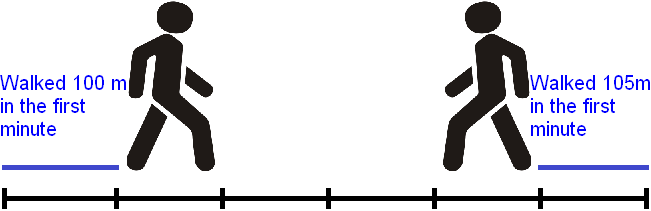
To find the speed of convergence, add the speeds of the objects.
Suppose that the pedestrians met three minutes after they started walking. Knowing that they met after three minutes, we can calculate the distance between the two points.
Every minute the pedestrians covered a distance equal to two hundred and five meters. After three minutes they met. So by multiplying speed sum by the time of movement, we can determine the distance between the two points:
205 × 3 = 615 meters.

You can also use another way to determine the distance between points. To do this, find the distance that each pedestrian walked before the meeting.
For example, the first pedestrian walked at a speed of 100 meters per minute. The meeting took place three minutes later, so in 3 minutes he walked 100 × 3 meters
100 × 3 = 300 meters.
And the second pedestrian walked at a speed of 105 meters per minute. In three minutes he walked 105 × 3 meters.
105 × 3 = 315 meters.
Now we can add up the results and determine the distance between the two points:
300 m + 315 m = 615 m
Task 1. Two cyclists simultaneously rode out of two settlements to meet each other. The speed of the first cyclist is 10 km/h, and the speed of the second cyclist is 12 km/h. They met after 2 hours of riding. Determine the distance between the two settlements.
Solution
Let's find the speed at which the cyclists approached
10 km/h + 12 km/h = 22 km/h.
Determine the distance between the settlements. To do this we multiply the closest approach speed by the travel time.
22 × 2 = 44 km

Solve this problem the second way. To do this, find the distances covered by each of them and add up the results.
Find the distance covered by the first cyclist:
10 × 2 = 20 km
Let's find the distance traveled by the second cyclist:
12 × 2 = 24 km
Add the distances obtained:
20 km + 24 km = 44 km
Answer: the distance between settlements is 44 km.
Task 2. Two cyclists simultaneously rode out of two settlements with 60 km distance between them to meet each other. The speed of the first cyclist is 14 km/h, and the speed of the second cyclist is 16 km/h. How many hours later did they meet?
Solution
Let's find the speed at which the cyclists approached each other:
14 km/h + 16 km/h = 30 km/h
In one hour the distance between the cyclists decreases by 30 km. To determine how many hours later they will meet, divide the distance between the two settlements by the speed of their approach:
60 : 30 = 2 hours
So the cyclists met after two hours.

Answer: The cyclists met after two hours.
Task 3. Two cyclists left from two settlements, the distance between which is 56 km, to meet each other at the same time. In two hours they met each other. The first cyclist rode at a speed of 12 km/h. Determine the speed of the second cyclist.
Solution
Determine the distance traveled by the first cyclist. Like the second cyclist, he spent two hours on the road. By multiplying the speed of the first bicycle rider by 2 hours, we can find out how many kilometers he rode before the meeting
12 × 2 = 24 km
In two hours, the first cyclist traveled 24 km. In one hour he walked 24:2, i.e., 12 km. Let's represent this graphically
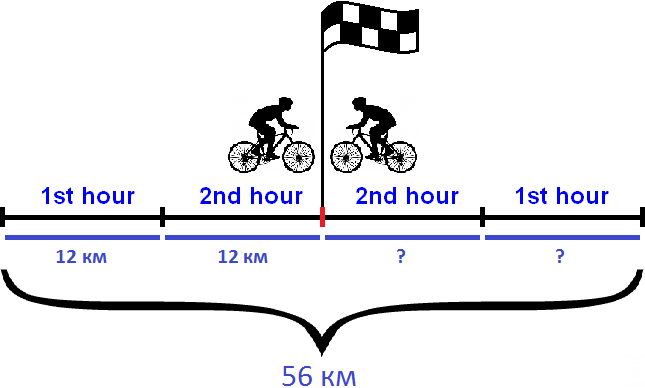
Subtract the distance covered by the first cyclist (24 km) from the total distance (56 km). This will determine how many kilometers the second cyclist covered:
56 km - 24 km = 32 km
The second cyclist, like the first cyclist, traveled for two hours. If we divide the distance he traveled by 2 hours, we find out at what speed he traveled:
32 : 2 = 16 km/h.
Therefore, the speed of the second cyclist is 16 km/h.
Answer: The speed of the second cyclist is 16 km/h.
Related Rates - Cars in Different Directions
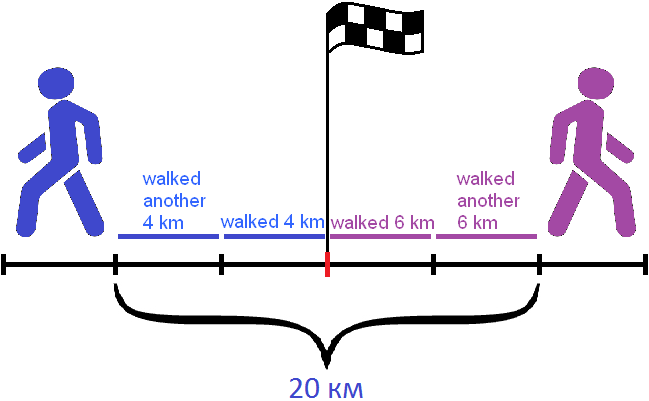
Lets take an example of two pedestrians that started walking from the same point in opposite directions, with the first pedestrian traveling at 4 km/h and the second at 6 km/h, then we add speeds (because they are going in opposite directions), which is 10 km/h.
Each hour the distance between the two pedestrians will increase by 10 kilometers.
To find the speed of two objects moving in opposite directions, add the speeds of the objects.
So in the first hour the distance between the pedestrians will be 10 kilometers. In the following figure you can see how this happens

You can see that the first pedestrian walked his 4 kilometers in the first hour. The second pedestrian also walked 6 kilometers in the first hour. In the first hour the distance between them was 4+6, i.e., 10 kilometers.
After two hours, the distance between the pedestrians will be 10×2, i.e., 20 kilometers. In the following figure you can see how this happens:
Task 1. A Freight Train and a Passenger Express departed from the same station simultaneously in opposite directions. The speed of the freight train was 40 km/h, the speed of the express train was 180 km/h. What will be the distance between these trains in 2 hours?
Solution
40 + 180 = 220 km/h.
This speed shows that in one hour the distance between the trains will increase by 220 km. In order to know what distance will be between the trains in two hours you should multiply 220 by 2.
220 × 2 = 440 km.
Answer: in two hours the distance between the trains will be 440 km.
Task 2. A bicyclist and a motorcyclist left the point simultaneously in opposite directions. The cyclist's speed is 16 km/h, and the motorcyclist's speed is 40 km/h. What will be the distance between the cyclist and the motorcyclist in 2 hours?
Solution
16 km/h + 40 km/h = 56 km/h.
This speed shows that in one hour the distance between a bicyclist and a motorcyclist will increase by 56 km.
Determine the distance that will be between the cyclist and the motorcyclist after 2 hours. To do this, multiply (56km/h) by 2 hours
56 × 2 = 112 km
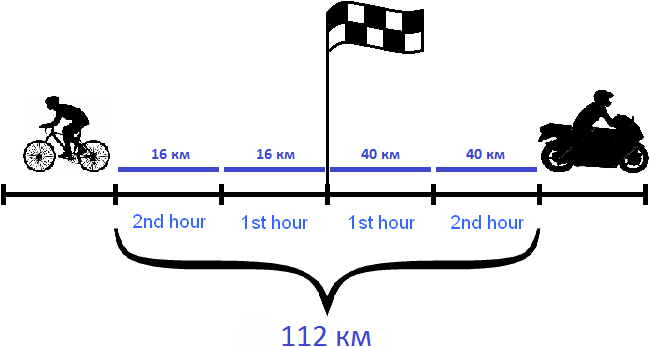
Answer: In 2 hours the distance between the cyclist and the motorcyclist will be 112 km.
Task 3. A bicyclist and a motorcyclist left the point simultaneously in opposite directions. The cyclist's speed is 10 km/h, and the motorcyclist's speed is 30 km/h. In how many hours it will be 80 km distance between them?
Solution
10 km/h + 30 km/h = 40 km/h.
In one hour, the distance between the cyclist and the motorcyclist increases by 40 km. To find out how many hours it will be 80 km distance between them, we have to determine how many times 80 km contains 40 km
80 : 40 = 2

Answer: 2 hours after the start of the movement, there will be 80 kilometers between the cyclist and the motorcyclist.
Task 4. A cyclist and a motorcyclist left the point simultaneously in opposite directions. After 2 hours the distance between them was 90 km. The speed of the cyclist was 15 km/h. Calculate the speed of the motorcyclist.
Solution
Determine the distance traveled by the cyclist in 2 hours. To do this, multiply his speed (15 km/h) by 2 hours
15 × 2 = 30 km
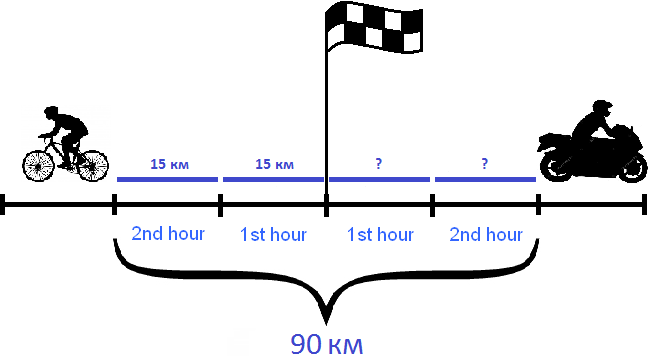
The figure shows that the cyclist is covering 15 kilometers each hour =>30 kilometers in two hours.
Let's subtract the distance covered by the cyclist (30 kilometers) from the total distance (90 kilometers). This will show us how many kilometers did the bicyclist travel:
90 km - 30 km = 60 km
The motorcyclist rode 60 km in two hours. If we divide the distance he traveled by two hours, we find out at what speed he traveled:
60 : 2 = 30 km/h.
So the speed of the motorcyclist was 30 km/h.
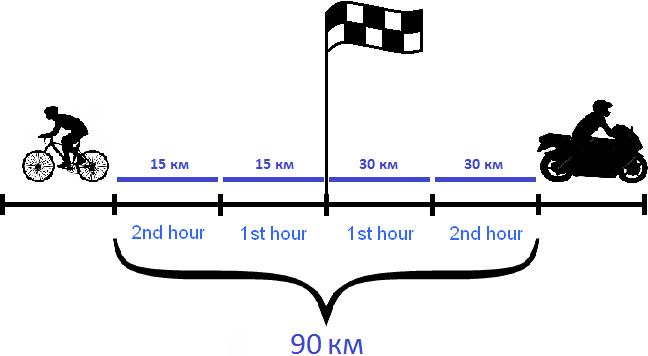
Answer: the speed of the motorcyclist was 30 km/h.
Task on the movement in one direction.
In the previous problem we considered problems in which objects (people, cars, boats) moved either towards each other or in opposite directions. We found different distances that varied between the objects over time.
In the first case, we found the related rates - in a situation where two objects were moving towards each other. In a unit of time, the distance between the objects decreased by a certain distance

In the second case, it was a situation where two objects were moving in opposite directions. In a unit of time, the distance between the objects increased by a certain distance

But objects can also move in the same direction, and at different speeds. For example, a cyclist and a motorcyclist can leave the same point at the same time, and the speed of the cyclist can be 20 kilometers per hour, and the speed of the motorcyclist - 40 kilometers per hour

The figure shows that the motorcyclist is twenty kilometers ahead of the cyclist. This is because he travels 20 kilometers more per hour than the cyclist. Therefore, every hour the distance between the cyclist and the motorcyclist will increase by twenty kilometers.
In this case, 20 km/h is the speed difference between motorcyclists and the cyclist.
After two hours, the distance traveled by the cyclist will be 40 km. The motorcyclist will cover 80 kilometers and will move another twenty kilometers away from the cyclist - a total distance of 40 kilometers between them.
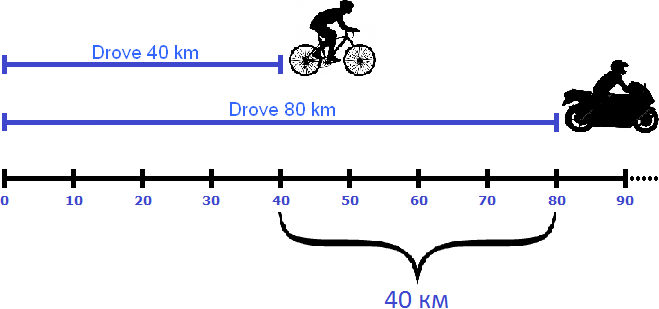
To find the speed of one object driving away from another when traveling in one direction, you must subtract the lower speed from the higher speed.
In the example above, this speed of one object diving away from another one is 20 km/h. It can be found by subtracting the speed of the cyclist from the speed of the motorcyclist. The speed of the cyclist was 20 km/h and the speed of the motorcyclist was 40 km/h. The motorcyclist's speed is greater, so we subtract 20 from 40
40 km/h - 20 km/h = 20 km/h
Task 1. A car and a bus left the city in the same direction. The speed of the car is 120 km/h, and the speed of the bus is 80 km/h. What will be the distance between them in 1 hour? 2 hours?
Solution
120 km/h - 80 km/h = 40 km/h.
Each hour the passenger car is driving 40 km away from the bus. In one hour the distance between the car and the bus will be 40 km. In 2 hours it is twice as much:
40 × 2 = 80 km
Answer: in one hour the distance between the car and the bus will be 40 km, in two hours it will be 80 km.
Consider a situation in which objects began their movement from different points, but in the same direction.
Suppose there is a house, a school, and an attraction. From the house to the school 700 meters
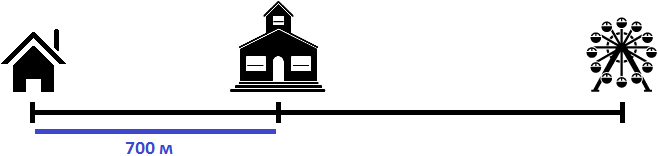
Task 6 Two pedestrians went on the ride at the same time. The first pedestrian went on the ride from home at 100 meters per minute, and the second pedestrian went on the ride from school at 80 meters per minute. What will be the distance between the pedestrians after 2 minutes? How many minutes after start will the first pedestrian catch up with the second pedestrian?
Let's answer the first question of the problem: What is the distance between the pedestrians after 2 minutes?
Determine the distance traveled by the first pedestrian in 2 minutes. He was moving at a speed of 100 meters per minute. In two minutes he will walk twice as much, i.e., 200 meters.
100 × 2 = 200 meters

Determine the distance traveled by the second pedestrian in 2 minutes. He moved at a speed of 80 meters per minute. In two minutes he will walk twice as much, i.e., 160 meters.
80 × 2 = 160 meters
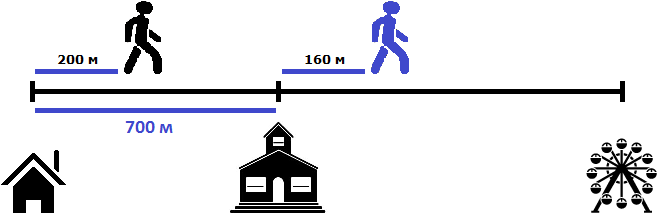
Now we need to find the distance between pedestrians
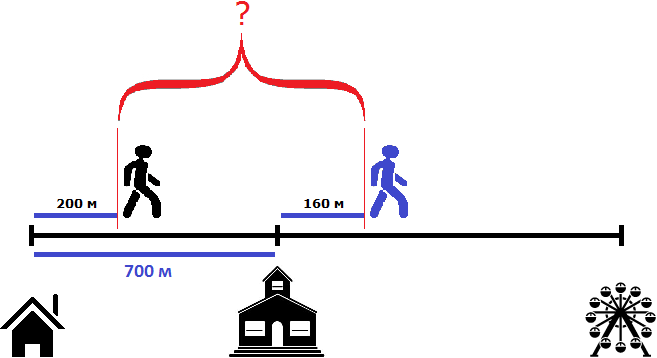
To find the distance between pedestrians, you can add the distance walked by the second pedestrian (160m) to the distance from home to school (700m) and subtract the distance walked by the first pedestrian (200m) from the result.
700 m + 160 m = 860 m
860 m - 200 m = 660 m
Either subtract the distance walked by the first pedestrian (200m) from the distance from home to school (700m) and add the distance walked by the second pedestrian (160m) to the result.
700 m - 200 m = 500 m
500 m + 160 m = 660 m
Thus, in two minutes the distance between pedestrians will be 660 meters

Let's try to answer the next question of the problem: How many minutes after the start of movement will the first pedestrian catch up with the second one?
Let's see what the situation was at the beginning of the journey-when the pedestrians had not yet begun their movement
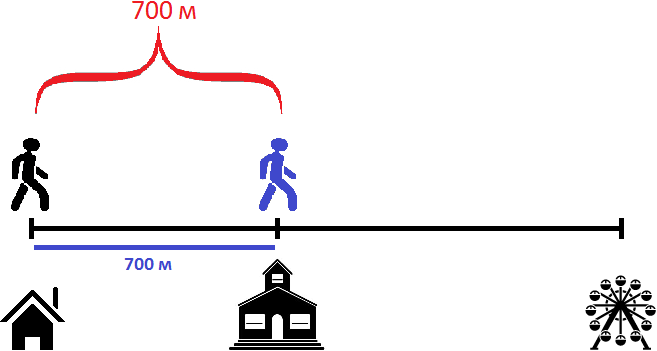
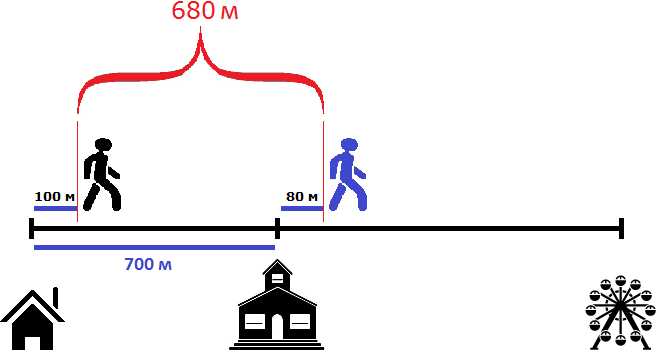
As you can see in the figure, the distance between the pedestrians at the beginning of the journey was 700 meters. But in a minute after the start of movement the distance between them will be 680 meters, because the first pedestrian is moving 20 meters faster than the second pedestrian:
100 m × 1 = 100 m
80 m × 1 = 80 m
700 m + 80 m - 100 m = 780 m - 100 m = 680 m
Two minutes after the start of the movement, the distance will decrease by another 20 meters and will be 660 meters. This was our answer to the first question of the problem:
100 m × 2 = 200 m
80 m × 2 = 160 m
700 m + 160 m - 200 m = 860 m - 200 m = 660 m

In three minutes the distance will decrease by another 20 meters and will already be 640 meters:
100 m × 3 = 300 m
80 m × 3 = 240 m
700 m + 240 m - 300 m = 940 m - 300 m = 640 m
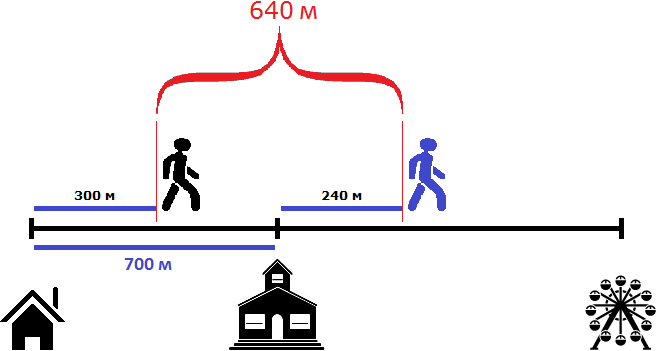
We see that with every minute the first pedestrian will approach the second pedestrian by 20 meters, and will eventually catch up with him. We can say that the speed of twenty meters per minute is the speed of convergence of the pedestrians. The rules for finding the speed of approaching and moving away in the same direction are identical.
To find the speed of convergence when traveling in the same direction, you must subtract the lower speed from the higher speed.
And since 700 meters decreases by the same 20 meters each minute, we can find out how many times 700 meters contains 20 meters each, thereby determining how many minutes later the first pedestrian will catch up with the second
700 : 20 = 35
So in 35 minutes after the start of the movement, the first pedestrian will catch up with the second pedestrian. For the sake of interest, find out how many meters each pedestrian has walked by this time. The first one was moving at a speed of 100 meters per minute. In 35 minutes he walked 35 times more
100 × 35 = 3500 m
The second walked at a speed of 80 meters per minute. In 35 minutes he walked 35 times more
80 × 35 = 2800 m
The first walked 3,500 meters and the second walked 2,800 meters. The first one walked 700 meters more, because he walked from home. If we subtract these 700 meters from 3,500 meters, we get 2,800 meters.
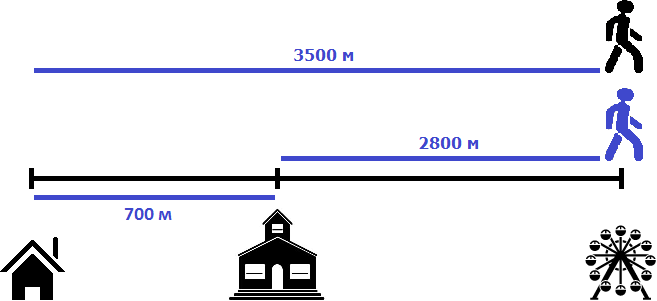
Task 7 Consider a situation in which objects are moving in the same direction, but one of the objects began its movement before the other.
Suppose there is a house and a school. The first pedestrian went to school at a speed of 80 meters per minute. After 5 minutes the second pedestrian followed him to school at a speed of 100 meters per minute. After how many minutes will the second pedestrian catch up with the first pedestrian?
The second pedestrian started walking 5 minutes later. By that time the first pedestrian had already covered some distance. Let's find this distance. For this purpose multiply his speed (80 m/m) by 5 minutes
80 × 5 = 400 meters

The first pedestrian is 400 meters away from the second pedestrian. Therefore, at the moment the second pedestrian begins his movement, there will be these 400 meters between them.
But the second pedestrian is moving at a speed of 100 meters per minute. That is, he moves 20 meters faster than the first pedestrian, which means that with every minute the distance between them will decrease by 20 meters. Our task is to find out after how many minutes this will happen.
For example, after one minute the distance between the pedestrians will be 380 meters. The first pedestrian will walk another 80 meters in addition to his 400 meters, and the second pedestrian will walk 100 meters.

The principle here is the same as in the previous problem. The distance between the pedestrians at the time of the second pedestrian's movement must be divided by the speed of the pedestrians' approach. The approaching speed in this case is twenty meters. Therefore, to determine how many minutes later the second pedestrian will catch up with the first pedestrian, divide 400 meters by 20
400 : 20 = 20
So in 20 minutes the second pedestrian will catch up with the first pedestrian.
Task 2. A bus and a cyclist left the two villages, the distance between which is 40 km, simultaneously in the same direction. The speed of the cyclist is 15 km/h, and the speed of the bus is 35 km/h. In how many hours will the bus catch up with the cyclist?
Solution
Find the speed of convergence
35 km/h - 15 km/h = 20 km/h.
How many hours will it take the bus to overtake the cyclist?
40 : 20 = 2
Answer: The bus will catch up with the cyclist in 2 hours.
River & boat & stream moving problem
Vessels move along the river at different speeds. They can move both downstream and upstream. Depending on how they move (upstream or downstream), the speed will change.
Suppose the speed of the river is 3 km/h. If you move the boat into the river, the river will carry the boat away at 3 km/h.
If you launch the boat into standing water, in which there is no stream, the boat will also be standing. The speed of the boat in this case will be zero.
If a boat is sailing in standing water with no current, the boat is said to be sailing at its own speed.
For example, if a motorboat is sailing in standing water at 40 km/h, then the motorboat's own speed is said to be 40 km/h.
How do I find the speed of a ship?
If the ship is sailing downstream, you must add the speed of the river to the ship's own speed.
For example, if a motorboat is sailing at 30 km/h along a river and the river stream speed is 2 km/h, then to the motorboat's own speed (30 km/h) you must add the speed of the river stream (2 km/h)
30 km/h + 2 km/h = 32 km/h
The river stream can be said to help the motorboat with an additional speed equal to two kilometers per hour.
If the ship sails against the flow of the river, you must subtract the speed of the flow of the river from the speed of the ship.
For example, if a motorboat sails at 30 km/h against the stream of a river, and the speed of the river stream is 2 km/h, then from the speed of the motorboat (30 km/h) you must subtract the speed of the river stream (2 km/h)
30 km/h - 2 km/h = 28 km/h
The river stream in this case prevents the motorboat from moving freely forward, reducing its speed by two kilometers per hour.
Task 1. The speed of the boat is 40 km/h, and the speed of the stream is 3 km/h. At what speed will the boat move downstream of the river? Against the flow of the river?
Answer:
If the boat were to move downstream of the river, its speed would be 40 + 3, which is 43 km/h.
If the boat moves against the stream of the river, its speed will be 40 - 3, i.e., 37 km/h.
Task 2. The speed of the motorboat in standing water is 23 km/h. The speed of the river stream is 3 km/h. What path will the ship take in 3 hours downstream of the river? Against the stream?
Solution
The actual speed of the ship is 23 km/h. If the ship moves downstream, its speed will be 23 + 3, i.e. 26 km/h. It will travel three times as much in three hours
26 × 3 = 78 km
If the ship moves against the river stream, her speed will be 23 - 3, i.e. 20 km/h. It will travel three times as much in three hours
20 × 3 = 60 km
Task 3. The boat covered the distance from point A to point B in 3 hours and 20 minutes, and the distance from point B to A in 2 hours and 50 minutes. In which direction is the river stream: from A to B or from B to A, if it is known that the speed of the boat didn't change during trip?
Solution
The speed of the boat did not change. Let us find out the direction of the river which took longer: the path from A to B or the path from B to A. The longest one is the path taken with the river stream against the boat
3 hours and 20 minutes more than 2 hours and 50 minutes. This means that the stream of the river reduced the speed of the boat and this was reflected in the travel time. 3 hours and 20 minutes is the time it took to go from A to B. So the river flows from point B to point A
Task 4. In what time when moving against the stream of the river the ship will run 204 km if its own speed 15 km/h, and the speed of the current is 5 times less than the speed of the ship speed?
Solution
Find the time it takes the ship to travel 204 kilometers against the stream of the river. The speed of the ship is 15 km/h. It is moving against the stream of the river, so we need to determine its speed in this motion.
To determine the speed against the stream of the river, subtract the speed of the ship (15 km/h) from its own speed (15 km/h). The condition states that the speed of the river stream is 5 times less than the speed of the boat, so first determine the speed of the river stream. To do this reduce 15 km/h by a factor of five
15 : 5 = 3 km/h
The speed of stream is 3 km/h. Let's subtract this speed from the speed of the boat
15 km/h - 3 km/h = 12 km/h.
Now determine the time needed for the ship to travel 204 km at 12 km/h. The ship travels 12 km per hour. To know how many hours it will pass 204 km, determine how many times 204 km contains 12 km
204 : 12 = 17 h
Answer: the ship will pass 204 kilometers in 17 hours
Task 5. Moving along the stream of the river, in 6 hours the boat
covered 102 km. Determine the boat's own speed,
if the speed of the stream is 4 km/h.
Solution
Find the speed at which the boat moved along the river. Divide the distance traveled (102 km) by the time of travel (6 h).
102 : 6 = 17 km/h.
Let us determine the boat's own speed. This is done by subtracting the speed of the stream (4 km/h) from the speed at which the boat traveled in the river (17 km/h)
17 - 4 = 13 km/h
Task 6. Moving against the stream, in 5 hours the boat
traveled 110 km. Determine the boat's own speed,
if the stream speed is 4 km/h.
Solution
Find the speed at which the boat moved along the stream. This is done by dividing the distance traveled (110 km) by the time of travel (5 h).
110 : 5 = 22 km/h.
Let us determine the boat's own speed. The condition states that the boat moved against the stream of the river. The speed of the river stream was 4 km/h. This means that the boat's own speed was reduced by 4. Our task is to add the 4 km/h and find the boat's own speed
22 + 4 = 26 km/h.
Answer: the boat's own speed is 26 km/h
Task 7. How long will it take for a boat moving against the stream of a river
would travel 56 km if the speed of the stream was 2 km/h and the boat's own speed was
own speed is 8 km/h more than the current speed?
Solution
Find the boat's own speed. The condition states that it is 8 km/h greater than the speed of the stream. Therefore let us add another 8 km/h to the stream speed (2 km/h) to find the boat's own speed
2 km/h + 8 km/h = 10 km/h
The boat is going against the stream of the river, so let's subtract the speed of the river (2 km/h) from the boat's own speed (10 km/h)
10 km/h - 2 km/h = 8 km/h
Find out how long it will take the boat to travel 56 km. For this we divide the distance (56 km) by the speed of the boat:
56 : 8 = 7 h
Answer: If the boat goes upstream, it will pass 56 km in 7 hours.
Exercises
Solution
In one hour a pedestrian walks 5 kilometers. To determine how long it takes him to walk 20 km, you need to find out how many times 20 kilometers contain 5 km. Or use the rule of finding time: divide the distance traveled by the speed of travel
20 : 5 = 4 hours
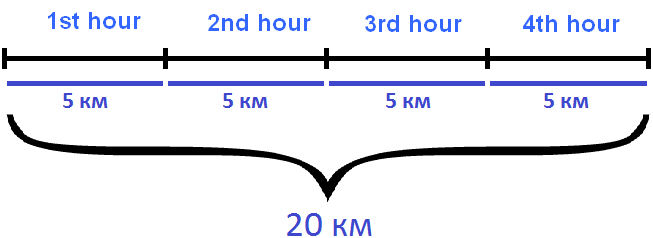
Solution
Determine the distance from point A to point B. To do this, multiply the speed at which the cyclist rode from point A to point B (16 km/h) by the travel time (5 h)
16 × 5 = 80 km
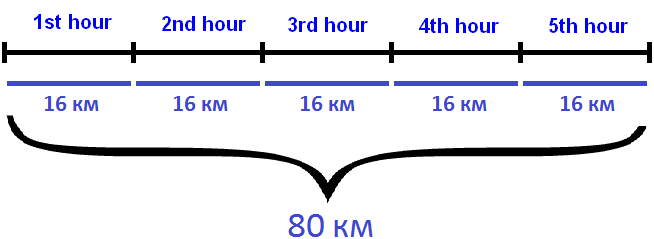
Find how much time the cyclist spent on the return trip. To do this, divide the distance (80 km) by the speed (10 km/h)
80 : 10 = 8 h
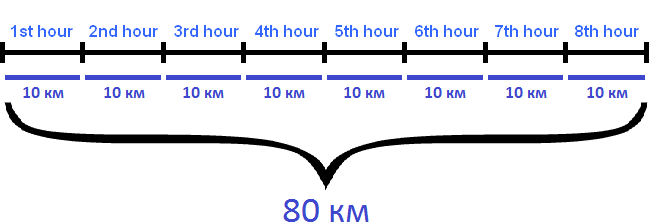
Solution
Find the distance covered by the cyclist in six hours. We subtract from 83 km the distance traveled after six hours (11 km).
83 - 11 = 72 km

Determine how fast the cyclist rode for the first 6 hours. To do this, divide 72 km by 6 hours
72 : 6 = 12 km/h
Since the condition of the problem is that the cyclist rode the remaining 11 km at the same speed he rode for the first 6 hours, the speed of 12 km/h is the answer to the problem.
Answer: the cyclist rode at a speed of 12 km/h.
Solution
Find the speed of flow of the river. The condition states that the raft can travel 72 kilometers in 36 hours. The raft cannot move against the flow of the river. So the speed at which the raft travels the 72 kilometers is the speed of the river flow. To find this speed, divide the 72 kilometers by 36 hours.
72 : 36 = 2 km/h
Let us find the speed of the boat. First we find her speed against the flow of the river. To do this we divide 72 kilometers by 4 hours
72 : 4 = 18 km/h
If the speed of the ship against the river flow is 18 km/h, then its own speed is 18+2, i.e. 20 km/h. And downstream of the river its speed is 20+2, i.e., 22 km/h
The ship's own speed is 20+2, i.e., 22 km/h.
Dividing the distance of 110 km by the speed of the ship in the river flow (22 km/h) you find out how many hours it takes the ship to travel the distance of 110 km
110 : 22 = 5 h
Answer: through the flow of the river the ship will travel 110 kilometers in 5 hours.
Solution
Find the removal speed of the cyclists
11 + 13 = 24 km
Find out the distance between them after 4 hours
24 × 4 = 96 km
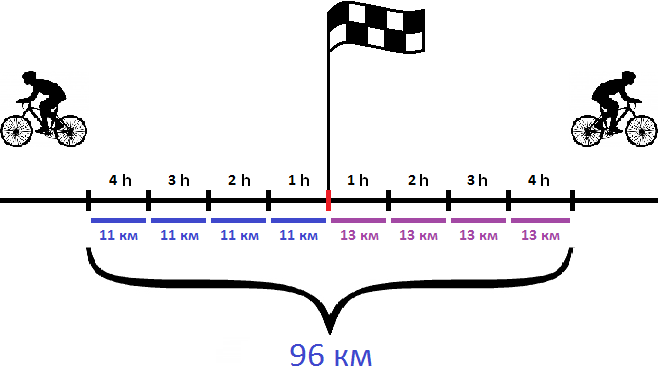
Answer: in 4 hours the distance between the cyclists will be 96 km.
Solution
Find the distance traveled by the first ship. For this purpose we multiply its speed (21 km/h) by the travel time before the meeting (6h).
21 × 6 = 126 km
Determine the distance travelled by the second ship. For this purpose multiply its speed (24 km/h) by the travel time before the meeting (6h)
24 × 6 = 144 km
Determine the distance between the piers. For this purpose we add the distances travelled by the first and the second vessels
126 km + 144 km = 270 km
Answer: the first ship covered 126 km, the second ship 144 km. The distance between the marinas is 270 km.
Solution
Find how many kilometers the train left New York before the meeting. To do this, multiply its speed (51 km/h) by 16 hours
51 × 16 = 816 km
Let's find out how many kilometers the train that left Orlando travelled before the meeting. To do this we subtract the distance travelled by the train that left New York City from the distance between New York City and Orlando (1,520 km).
1520 - 816 = 704 km
Find the speed at which the train left Orlando. To do this, divide the distance it traveled before the meeting by 16 hours
704 : 16 = 44 km/h
Let's find the distance that will be between the trains 5 hours after they meet. To do this, find the speed of the trains and multiply that speed by 5
51 km/h + 44 km/h = 95 km/h
95 × 5 = 475 km.
Answer: the train that left Orlando was traveling at 44 km/h. Five hours after the trains met, the distance between them would be 475 km.
Solution
Find the speed of the second bus. It is 6 km/h more than the speed of the first bus
48 km/h + 6 km/h = 54 km/h
Find the speed of removal of the buses. To do this, add their speeds:
48 km/h + 54 km/h = 102 km/h
In one hour the distance between the buses increases by 102 kilometers. To find out after how many hours the distance between them will be 510 km, we need to find out how many times 510 km contains 102 km/h
510 : 102 = 5 h
An answer: 510 km between the buses will be in 5 hours.
Solution
Let's find the speed of the Chicago train.It is ![]() of the speed of the New York train. So to determine the speed of the Chicago train, we need to find
of the speed of the New York train. So to determine the speed of the Chicago train, we need to find ![]() of 63 km
of 63 km
63 : 21 × 20 = 3 × 20 = 60 km/h
Let's find the closing speed of the trains
63 km/h + 60 km/h = 123 km/h
Determine after how many hours the trains will meet
1230 : 123 = 10 hrs
Let's find the distance at which the trains will meet from Chicago.To do this, just find the distance traveled by the Chicago train before meeting
60 × 10 = 600 km.
Answer: the trains will meet at a distance of 600 km from Chicago.
Solution
Find the speed of the second boat. It is 75% of the speed of the first boat. Therefore, to find the speed of the second boat we need 75% of 16 km
16 × 0.75 = 12 km/h
Find the speed of convergence of the boats
16 km/h + 12 km/h = 28 km/h
The distance between the boats will decrease by 28 km every hour. After 2 hours it would be 28×2, i.e. 56 km. In order to determine the distance between the boats at that time we subtract 56 km from 75 km
75 km - 56 km = 19 km
Answer: two hours later there will be 19 km between the boats.
Solution

Find the speed of approach
62 km/h - 47 km/h = 15 km/h
If initially the distance between the cars was 60 km, then every hour this distance will decrease by 15 km, and eventually the passenger car will catch up with the truck. To find out how many hours later this will happen, determine how many times 60 km contains 15 km
60 : 15 = 4 h
Let's find out at what distance from the beginning of the movement the passenger car caught up with the truck. To do this we multiply the speed of the car (62 km/h) by the time of its movement before the meeting (4 h)
62 × 4 = 248 km
Answer: the passenger car will catch up with the truck in 4 hours. At the time of the meeting the passenger car will be 248 km away from the start of the movement.
Solution
Find the speed of the second motorcyclist. It is 80% of the speed of the first motorcyclist. Therefore, to find the speed of the second motorcyclist, we need to find 80% of 35 km/h
35 × 0.80 = 28 km/h
The first motorcyclist is moving 35-28 km/h fast
35 km/h - 28 km/h = 7 km/h
In one hour, the first motorcyclist travels 7 kilometers more. With each hour it will get closer to the second motorcyclist by those 7 kilometers.
In 5 hours, the first motorcyclist will have covered 35×5, i.e. 175 km, and the second motorcyclist will have covered 28×5, i.e. 140 km. Let's determine the distance that is between them. To do this, we subtract 140 km from 175 km
175 - 140 = 35 km
Answer: in 5 hours the distance between the motorcyclists will be 35 km.
Solution

Find the speed of approach:
43 km/h - 13 km/h = 30 km/h
If initially the distance between the motorcyclist and the cyclist was 120 km, then every hour this distance will decrease by 30 km, and eventually the motorcyclist will catch up with the cyclist. To find out how many hours later this will happen, determine how many times 120 km contains 30 km
120 : 30 = 4 h
So in 4 hours the motorcyclist will catch up with the cyclist

The figure shows the movement of a motorcyclist and a bicyclist. You can see that after 4 hours of driving, they have aligned.
Answer: the motorcyclist will catch up with the cyclist after 4 hours.
Solution
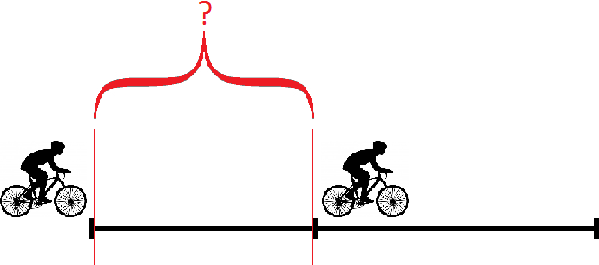
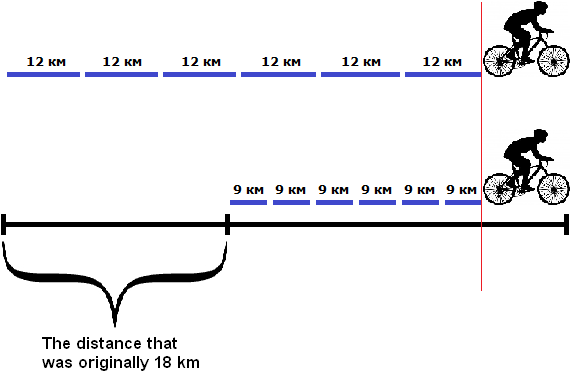
Find the speed of the cyclist riding in front. To do this, find 75% of the speed of the cyclist riding behind:
12 × 0.75 = 9 km/h is the speed of the cyclist riding in front
Let's find out how many kilometers each cyclist rode before the second cyclist caught up with the first one:
12 × 6 = 72 km - the one riding behind
9 × 6 = 54 km - the one riding in front
Let's find out the initial distance between the cyclists. To do this, we subtract the distance traveled by the second cyclist (who was catching up) from the distance traveled by the first cyclist (who was catching up)
72 km - 54 km = 18 km
Solution

Find the speed of the car away from the bus
53 km/h - 41 km/h = 12 km/h
The car will move 12 km away from the bus every hour. The figure shows the position of the cars after the first hour
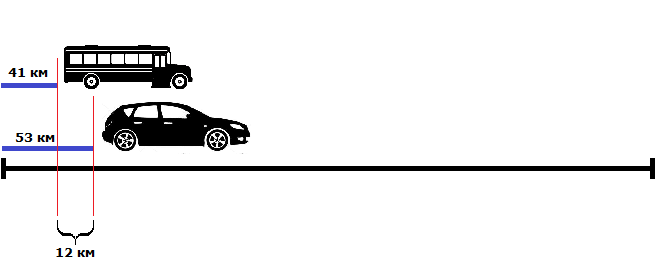
You can see that the car is 12 km ahead of the bus.
To find out in how many hours the car will be 48 km ahead of the bus, you have to determine how many times 48 km contains 12 km
48 : 12 = 4 h
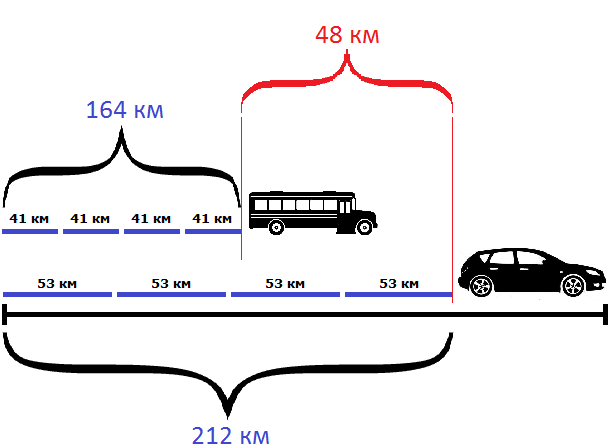
Answer: 4 hours after leaving, the car will be 48 kilometers ahead of the bus.
2. If you find an error or inaccuracy, please describe it.
3. Positive feedback is welcome.