Subtracting mixed numbers

There are problems where you need to subtract one mixed number from another mixed number. For example, find the value of the expression:

To solve this example, convert the mixed numbers and to improper fractions, then subtract fractions with different denominators:

If you subtract two whole pizzas and a third of a pizza from three whole pizzas, you are left with one whole pizza and one sixth of a pizza:

Example 2. Find the value of the expression

Convert the mixed numbers and into improper fractions and subtract fractions with different denominators:

We'll come back to the subtraction of mixed numbers. There are many subtleties in subtracting fractions that a beginner is not yet prepared for. For example, it is possible that the subtractor may be less than the subtractor. This can lead us into the world of negative numbers, which we haven't yet studied.

In the meantime, we will study multiplication of mixed numbers. It is not as complicated as addition and subtraction.

Subtraction of mixed numbers. Complex cases.

When subtracting mixed numbers, you sometimes find that things don't go as smoothly as you'd like. It often happens that when you solve an example, the answer is not what it should be.

When subtracting numbers, the subtractor must be greater than the subtractor. Only then will you get a normal answer.

For example, 10-8=2

10 is the diminutive

8 - subtraction

2 - difference

The diminutive 10 is greater than the subtractor 8, so we have a normal answer of 2.

Now let's see what happens if the subtractor is less than the subtractor. Example 5-7= -2

5 - diminutive

7 - subtraction

-2 - difference

In this case, we go beyond our usual numbers and enter the world of negative numbers, where it is too early, or even dangerous, for us to walk. To work with negative numbers, you need proper mathematical training, which we have not yet received.

If when solving subtraction examples you find that the subtractor is smaller than the subtractor, you can skip such an example for now. It is acceptable to work with negative numbers only after you have studied them.

The situation with fractions is the same. The diminutive must be greater than the subtractor. Only then will it be possible to get a normal answer. And to know if the fraction to be reduced is larger than the fraction to be subtracted, you need to be able to compare the fractions.

For example, solve example.

This is a subtraction example. To solve it, you must check to see if the fraction being subtracted is greater than the fraction being subtracted. is more than

so we can safely go back to the example and solve it:

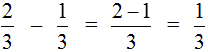
Now solve this example

Check to see if the fraction to be subtracted is larger than the fraction to be subtracted. We find that it is smaller:

In this case, it is wise to stop and not continue with further calculations. We will return to this example when we study negative numbers.

It is also desirable to check mixed numbers before subtraction. For example, find the value of the expression .

First, we check to see if the decreasing mixed number is greater than the subtracted one. To do this, convert the mixed numbers into improper fractions:

We got fractions with different numerators and different denominators. To compare such fractions, we need to reduce them to the same (common) denominator. We will not describe in detail how to do it. If you have difficulties, be sure to repeat the actions with fractions.

After reducing the fractions to the same denominator, we get the following expression:

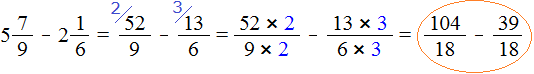
Now we need to compare fractions and . These are fractions with the same denominators. Of the two fractions with the same denominators, the fraction whose numerator is larger is larger.

The fraction has a larger numerator than the fraction . So the fraction is larger than the fraction .

This means that the subtractor is greater than the subtractor .

Which means we can go back to our example and boldly solve it:

Example 3. Find the value of the expression

Check to see if the subtractor is greater than the subtractor.

Convert the mixed numbers into improper fractions:

We obtained fractions with different numerators and different denominators. Bring these fractions to the same (common) denominator: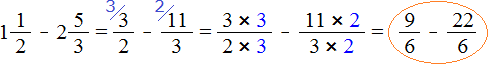

Now let's compare fractions and . The fraction has a smaller numerator than the fraction , so the fraction is smaller than the fraction

This means that the diminutive of is also less than the subtractor of

This is guaranteed to lead us into the world of negative numbers. So it makes more sense to stop here and not continue calculating. We'll continue when we study negative numbers.

Example 4. Find the value of the expression

Check to see if the subtractor is greater than the subtractor.

Convert the mixed numbers into improper fractions:

We got fractions with different numerators and different denominators. Bring them to the same (common) denominator:

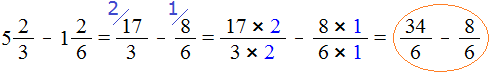
Now we need to compare fractions and . The fraction has a larger numerator than the fraction . So the fraction is larger than the fraction .

This means that the subtractor is greater than the subtractor .

Therefore, we can safely continue to calculate our example:

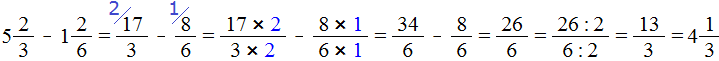
At first we got the answer . We reduced this fraction by 2 and got a fraction , but we were not satisfied with this answer either, so we separated the whole part in this answer. As a result, we got the answer .

Video lesson

Add comment