Dividing a smaller number by a larger number

Situations often arise in life when you need to divide a smaller number by a larger one. For example, imagine a situation. There are three friends:

And you need to divide the two apples equally between them. How to do this? There are three friends and only two apples. We find ourselves in a situation where we need to divide a smaller number by a larger one (two apples for three).

The following rule is provided for such cases:

Dividing a smaller number by a larger number produces a fraction with the smaller-one divisor in the numerator and the bigger-one divisor in the denominator.

Let's apply this rule. It says that dividing a smaller number by a larger number produces a fraction with the numerator being the divisor and the denominator being the divisor. The divisor is two apples. Write the number 2 in the numerator:

And the divisor is the three friends (remember, the divisor shows how many parts we need to divide the divisor into). Write the three in the denominator of our fraction:

It's funny, but a fraction of is the answer to our problem. Each friend gets of an apple. Why did this happen?

To divide two apples among three people, you must cut each apple with a knife into three pieces and distribute these pieces equally among the three friends:

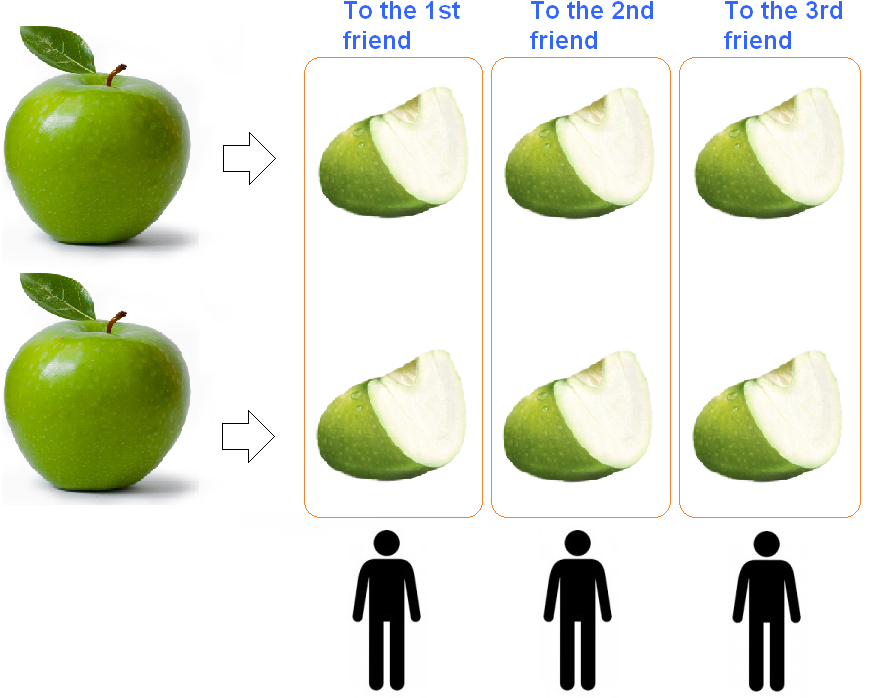
As you can see in the picture, each apple was divided into three pieces and scattered equally among the three friends. Each friend got of an apple (two slices out of three).

Dividing a smaller number by a larger number. Advanced level.

In a previous lesson, we said that dividing a smaller number by a larger number produces a fraction with the numerator being the smaller-one and the denominator being the bigger-one.

For example, to divide one apple by two, you need to write 1 (one apple) in the numerator and 2 (two friends) in the denominator. The result is a fraction of . So each friend gets of an apple. In other words, half an apple. The fraction is the answer to the problem "how to divide an apple between two people".

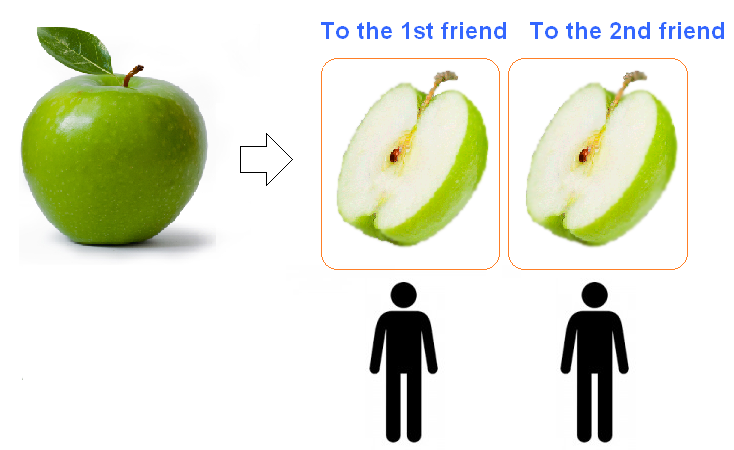
It turns out that you can solve this problem further by dividing 1 by 2. After all, the fractional line in any fraction means division, and therefore in fractions this division is allowed. But how? We are used to the fact that the dividend is always greater than the divisor. But here it is the opposite, the dividend is less than the divisor.

Everything becomes clear if you remember that fraction means partition, division And so a unit can be divided into as many parts as you want, not just two parts.

Dividing a smaller number by a larger number produces a decimal in which the whole part is 0 (zero). The fractional part can be anything.

So, divide 1 by 2. Solve this example with a long division (column division):

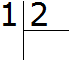
You can't just divide one by two by an whole. If you ask the question "how many twos are in one", the answer is 0. So we write 0 in the quotient and dot it:

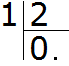
Now multiply the quotient by the divisor, as usual, to take out the remainder:

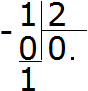
Now is the moment when the one can be divided into two parts. To do this, add another zero to the right of the resulting one:

We got 10. We divide 10 by 2 and get 5. Write 5 on the fractional part of our answer:

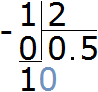
Now pull out the last remainder to complete the calculation. Multiply 5 by 2 to get 10

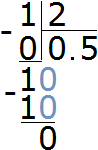
The answer is 0.5. So fraction equals 0.5

Half of an apple can also be written with the decimal 0.5. If we add the two halves (0.5 and 0.5), we again get the original one whole apple:

This point can also be understood by imagining how 1 cm is divided into two parts. If you divide 1 cm by 2 parts, you get 0.5 cm

Example 2. Find the value of the expression 4 : 5

How many fives are in a four? None. Write 0 in the quotient and put a decimal point in it:

We multiply 0 by 5, we get 0. We write zero under the four. Immediately subtract that zero from the divisor:

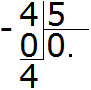
Now we begin to divide (divide) the four into 5 parts. To do this, add a zero to the right of 4 and divide 40 by 5 to make 8. Write the eight in the quotient.

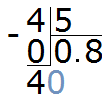
Complete the example by multiplying 8 by 5 to get 40:

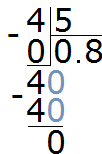
The answer is 0.8. So the value of the expression 4 : 5 is 0.8

Example 3. Find the value of the expression 5 : 125

How many numbers are 125 in five? None. Write 0 in the quotient and dot it:

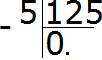
Multiply 0 by 125 to get 0. Write 0 under the five. Immediately subtract 0 from the five.

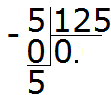
Now let's start splitting the five into 125 parts. To do this, write a zero on the right side of this five:

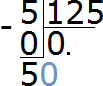
Divide 50 by 125. How many numbers are 125 in the number 50? None. So we again write 0 in the quotient.

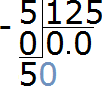
We multiply 0 by 125, we get 0. We write that zero under 50. Immediately subtract 0 from 50.

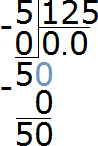
Now divide the number 50 into 125 parts. To do this, write another zero to the right of 50:

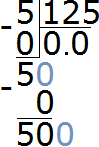
Divide 500 by 125. How many numbers 125 are in the number 500. There are four numbers 125 in the number 500. Write the four in the quotient:

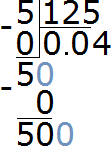
Complete the example by multiplying 4 by 125 to get 500

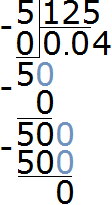
The answer is 0.04. So the value of the expression 5 : 125 is 0.04

Video lesson

Add comment