Adding fractions with common denominator

To add fractions with the same denominators, add their numerators and leave the denominator unchanged.

For example, let's add fractions and . Add up the numerators and leave the denominator unchanged:

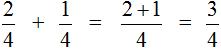
This example can be easily understood if you think of a pizza that is divided into four parts. If you add pizzas to pizza, you get pizza:

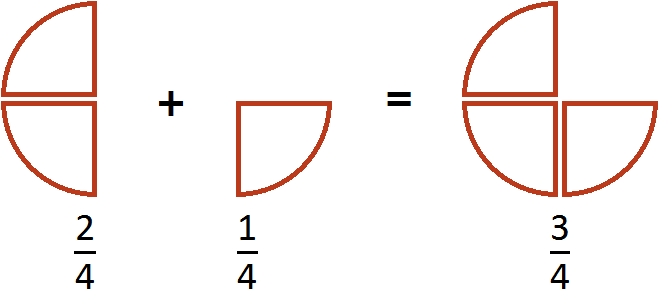
Example 2. Add fractions and .

Again we add the numerators and leave the denominator unchanged:

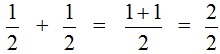
The answer is an incorrect fraction.. If the end of the problem comes, it is customary to get rid of incorrect fractions. To get rid of an irregular fraction, you need to select the integer part of it. In our case, the integer part is easy - two divided by two is one:

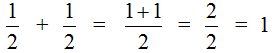
This example can be easily understood if you think of a pizza that is divided into two parts. If you add another pizza to the pizza, you get one whole pizza:

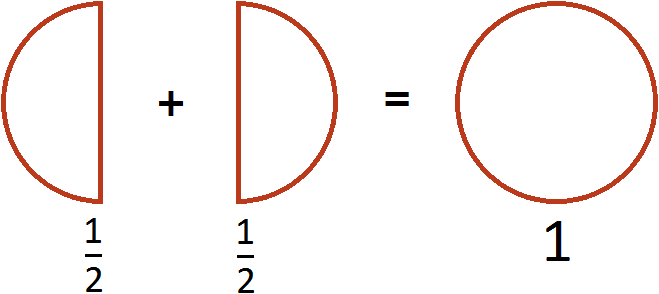
Example 3. Add fractions and .

Again we add the numerators and leave the denominator unchanged:

This example can be easily understood if you think of a pizza that is divided into three parts. If you add another pizza to pizza, you get pizza:

Example 4. Find the value of the expression

This example is solved in the same way as the previous ones. The numerators must be added and the denominator left unchanged:

Let's try to represent our solution with a picture. If you add pizza to pizza and add another pizza, you get 1 whole pizza and another pizza.

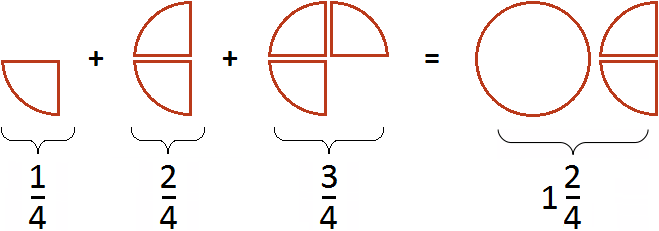
As you can see, there is nothing complicated about adding fractions with equal denominators. It is enough to understand the following rules:

1. To add fractions with the same denominators, add their numerators and leave the denominator unchanged;
2. If the answer is an improper fraction, you need to select the integer part of it.

Exercises:

Task 1. Find the value of the expression:

Solution:

Show Solution

Task 2. Find the value of the expression:

Solution:

Show Solution

Video lesson

Add comment