What is a quadratic equation and how do you solve it?
We remember that an equation is an equality that contains a variable whose value must be found.
If the variable in the equation is raised to the second power (squared), then such an equation is called a second-power equation or a quadratic equation.
For example, the following equations are quadratic:
Solve the first of these equations, namely x2 − 4 = 0.
All of the identity transformations that we applied when solving ordinary linear equations can also be applied when solving quadratic equations.
So, in the equation x2 - 4 = 0, move the term -4 from the left side to the right side, changing the sign:
We obtained the equation x2 = 4. Earlier we said that an equation is considered solved if one part of the variable is written in the first power and its coefficient is equal to one, and the other part is equal to some number. That is, to solve an equation, it must be reduced to the form x = a, where a is the root of the equation.
We still have the variable x in the second power, so the solution must be continued.
To solve the equation x2 = 4, you need to answer the question at what value of x the left part becomes equal to 4. Obviously, at values of 2 and -2. To derive these values, use the definition of the square root.
A number b is called the square root of a if b2 = a and is denoted as 
We have a similar situation right now. After all, what is x2 = 4? The variable x in this case is the square root of 4, because the second power of x is equal to 4.
Then we can write that 
. Calculating the right hand side will tell you what x is equal to. The square root has two values: positive and negative. Then we obtain x = 2 and x = -2.
The usual way to write it is to put a plus or minus sign in front of the square root and then find the arithmetic value of the square root. In this case, when is written, 
must be preceded by ±
Then find the arithmetic value of the square root
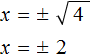
The expression x = ± 2 means that x = 2 and x = -2. That is, the roots of the equation x2 - 4 = 0 are 2 and -2. Let's write down the solution to this equation in full:
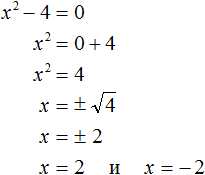
Let's check. Substitute the roots 2 and -2 in the original equation and perform the corresponding calculations. If at 2 and -2 the left side equals zero, it means that the equation is solved correctly:
In both cases the left-hand side is zero. So the equation is solved correctly.
Let's solve one more equation. Suppose we need to solve the quadratic equation (x + 2)2 = 25
First, let's analyze this equation. The left side is squared and it is 25. What number squared is 25? Obviously, the numbers 5 and -5
That is, our problem is to find x at which the expression x + 2 is equal to the numbers 5 and -5. Let's write down these two equations:
Let's solve both equations. These are ordinary linear equations that are easy to solve:
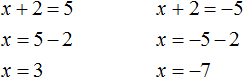
So the roots of the equation (x + 2)2 = 25 are the numbers 3 and -7.
In this example, as in the past, you can use the definition of a square root. Thus, in the equation (x + 2)2 = 25, the expression (x + 2) is the square root of 25. Therefore, we can first write that 
.
Then the right side of the equation is ±5. We get two equations: x + 2 = 5 and x + 2 = -5. Solving each of these equations separately, we arrive at the roots of 3 and -7.
Write down in full the solution of the equation (x + 2)2 = 25
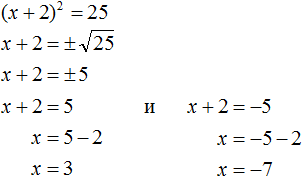
You can see from the examples above that the quadratic equation has two roots. In order not to forget about the found roots, the variable x can be signed with lower indices. For example, the root of 3 can be denoted by x1, and the root of -7 by x2.
In the previous example you could also do this. The equation x2 - 4 = 0 had roots 2 and -2. These roots could be designated as x1 = 2 and x2 = -2.
It also happens that a quadratic equation has only one root or no roots at all. We will look at such equations later.
Let's check for the equation (x + 2)2 = 25. Substitute the roots of 3 and -7 into it. If at 3 and -7 the left hand side equals 25, it means that the equation is solved correctly:
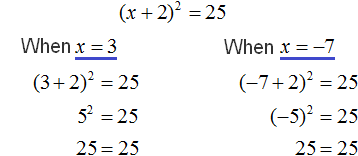
In both cases, the left-hand side is 25. So the equation is solved correctly.
A quadratic equation can be given in different forms. The most common form is as follows:
ax2 + bx + c = 0,
where a, b, c are some numbers, x is an unknown.
This is the so-called general form of the quadratic equation. In such an equation, all the terms are gathered in a common place (in one part), and the other part is zero. Otherwise, this kind of equation is called the normal kind of quadratic equation.
Suppose the equation is 3x2 + 2x = 16. In it, the variable x is raised to the second power, so the equation is quadratic. Let us reduce this equation to the general form.
So we need to get an equation that will be similar to the equation ax2 + bx + c = 0. To do this in the equation 3x2 + 2x = 16, move 16 from the right side to the left side, changing the sign:
3x2 + 2x − 16 = 0
We obtain the equation 3x2 + 2x - 16 = 0. In this equation a = 3, b = 2, c = -16.
In a quadratic equation of the form ax2 + bx + c = 0, the numbers a, b, and c have their own names. Thus, the number a is called the first or highest coefficient; the number b is called the second coefficient; the number c is called the free term.
In our case, for the equation 3x2 + 2x - 16 = 0, the first or highest coefficient is 3; the second coefficient is the number 2; the free term is the number -16. There is another common name for the numbers a, b, and c - parameters.
So, in the equation 3x2 + 2x - 16 = 0, the parameters are the numbers 3, 2, and -16.
In a quadratic equation, it is desirable to arrange the terms so that they are in the same order as the normal form of the quadratic equation.
For example, if the equation −5 + 4x2 + x = 0 is given, it is desirable to write it in normal form, that is, in the form ax2+ bx + c = 0.
In the equation −5 + 4x2 + x = 0 you can see that the free term is -5, it should be located at the end of the left part. The term 4x2 contains the higher coefficient, it should be placed first. The term x will be placed second accordingly:
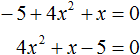
The quadratic equation can take a different form depending on the case. It all depends on what the values of a, b, and c are equal to.
If the coefficients a, b, and c are not zero, then the quadratic equation is called complete. For example, the quadratic equation 2x2 + 6x - 8 = 0 is complete.
If any of the coefficients is zero (i.e., absent), the equation is greatly reduced and takes a simpler form. Such a quadratic equation is called an incomplete. For example, a quadratic equation 2x2 + 6x = 0 is incomplete; it has coefficients a and b (numbers 2 and 6), but no free term c.
Let's look at each of these types of equations, and for each of these types let's determine a different way to solve them.
Let the quadratic equation 2x2 + 6x - 8 = 0 be given. In this equation a = 2, b = 6, c = -8. If we make b equal to zero, the equation will take the form:
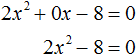
We obtain the equation 2x2 - 8 = 0. To solve it, move -8 to the right side, changing the sign:
2x2 = 8
To further simplify the equation we will use the previously studied identity transformations. In this case we can divide both parts by 2
We have the equation we solved at the beginning of this lesson. To solve the equation x2 = 4, use the definition of the square root. If x2 = 4, then 
. Hence x = 2 and x = -2.
So the roots of the equation 2x2 - 8 = 0 are the numbers 2 and -2. Let us write down in full the solution of this equation:
Let's check. Substitute the roots 2 and -2 in the original equation and perform the corresponding calculations. If at 2 and -2 the left side equals zero, it means that the equation is solved correctly:
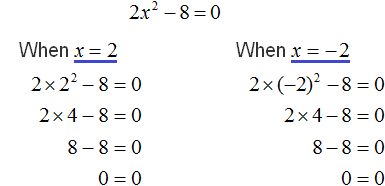
In both cases the left side is zero, so the equation is solved correctly.
The equation we just solved is an incomplete quadratic equation. If a complete quadratic equation looks like ax2 + bx + c = 0, then making the coefficient of b zero will make the incomplete quadratic equation ax2 + c = 0.
At first we also had a full quadratic equation 2x2 + 6x - 4 = 0. But we made the coefficient of b zero, that is, instead of number 6 we put 0. As a result, the equation turned into an incomplete quadratic equation 2x2 - 4 = 0.
At the beginning of this lesson, we solved the quadratic equation x2 - 4 = 0. This equation is also an equation of the form ax2 + c = 0, that is, it is incomplete. It has a = 1, b = 0, and c = -4.
Also, a quadratic equation is incomplete if the coefficient of c is zero.
Consider the full quadratic equation 2x2 + 6x - 4 = 0. Let's make the coefficient c zero. That is, instead of number 4 we put 0
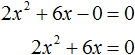
We obtain a quadratic equation 2x2 + 6x=0, which is incomplete. To solve such an equation, the variable x is taken out of brackets:
We obtain the equation x(2x + 6) = 0 in which we need to find x, at which the left part becomes equal to zero. Note that in this equation, the expressions x and (2x + 6) are the factors. One of the properties of multiplication says that a product is zero if at least one of the factors is zero (either the first factor or the second factor).
In our case, the equality will be achieved if x equals zero or (2x + 6) equals zero. So let's write it that way to begin with:
We have two equations: x = 0 and 2x + 6 = 0. The first equation does not need to be solved - it is already solved. That is, the first root is zero.
To find the second root, solve the equation 2x + 6 = 0. This is an ordinary linear equation that is easy to solve:
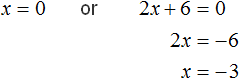
We see that the second root is -3.
So the roots of the equation 2x2 + 6x = 0 are 0 and -3. Let us write down in full the solution of this equation:
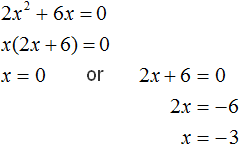
Let's check. Substitute the roots 0 and -3 in the original equation and perform the corresponding calculations. If at values 0 and -3 the left-hand side equals zero, it means that the equation is solved correctly:
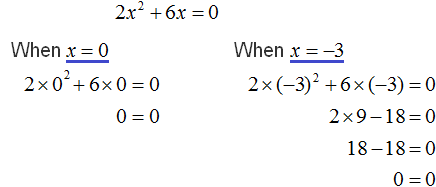
The next case is when the numbers b and c are zero. Consider the complete quadratic equation 2x2 + 6x - 4 = 0. Let's make the coefficients b and c zero. Then the equation will take the form:
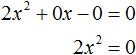
We obtain the equation 2x2 = 0. The left side is the product, and the right side is zero. A product is zero if at least one of the factors is zero. Obviously, x = 0. Indeed, 2 × 02 = 0. Hence, 0 = 0. For other values of x the equality will not be achieved.
Simply put, if in a quadratic equation of the form ax2 + bx + c = 0 the numbers b and c are zero, then the root of such an equation is zero.
Note that when the phrases "b equals zero" or "c equals zero" are used, it is implied that the parameters b or c are not in the equation at all.
For example, if the equation 2x2 - 32 = 0 is given, then we say that b = 0. Because if we compare with the full equation ax2 + bx + c = 0, we can see that in the equation 2x2 - 32 = 0 there is a major coefficient a, equal to 2; there is a free term -32; but there is no coefficient b.
Finally, consider the complete quadratic equation ax2 + bx + c = 0. As an example, solve the quadratic equation x2 - 2x + 1 = 0.
So, we need to find x, at which the left-hand side equals zero. Let us use the identity transformations we studied earlier.
First of all, notice that the left side of the equation is the square of the difference of two expressions. If we remember how to decompose a polynomial into factors, we get (x - 1)2 on the left side.
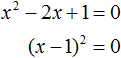
Let's reason further. The left part is squared and it is equal to zero. What number squared equals zero? Obviously, only 0. So our problem is to find x at which x - 1 is zero. By solving the simplest equation x - 1 = 0, we can find what x equals
The same result can be obtained by using the square root. In the equation (x - 1)2 = 0, the expression (x - 1) is the square root of zero. Then we can write that 
. In this example, it is not necessary to write ± in front of the root because the root of zero has only one value, zero. Thus x - 1 = 0. Hence x = 1.
So the root of the equation x2 - 2x + 1 = 0 is one. This equation has no other roots. In this case, we solved a quadratic equation that has only one root. It also happens.
Simple equations are not always given. Consider for example the equation x2 + 2x - 3 = 0.
In this case, the left part is no longer the square of the sum or the difference. So we need to look for other ways to solve this problem.
Note that the left part of the equation is a quadratic trinomial. Then we can try to extract the full square from this trinomial and see what it gives us.
Let's extract the full square from the quadratic trinomial, which is located on the left side of the equation:
In the resulting equation, move -4 to the right side, changing the sign:
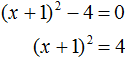
Now let's use the square root. In the equation (x + 1)2 = 4, the expression (x + 1) is the square root of 4. Then we can write that 
. Calculating the right hand side will give the expression x + 1 = ±2. From this we get two equations: x + 1 = 2 and x + 1 = -2, whose roots are the numbers 1 and -3
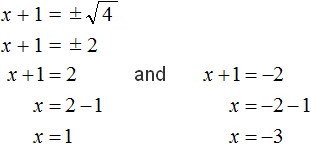
So the roots of the equation x2 + 2x - 3 = 0 are the numbers 1 and -3.
Let's make a check:
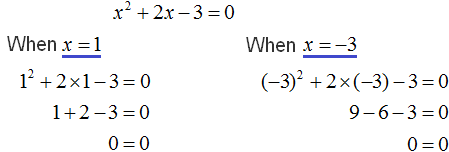
Example 3. Solve the equation x2 - 6x + 9 = 0 by singling the complete square.
Let's select the full square from the left part:
Next, use the square root and find out what x equals
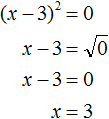
So the root of the equation x2 - 6x + 9 = 0 is 3. Let's check it:
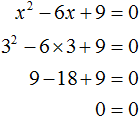
Example 4. Solve the quadratic equation 4x2 + 28x - 72 = 0 by singling the complete square:
Let's select the full square from the left part:
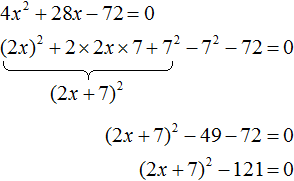
Move -121 from the left part to the right part, changing the sign:
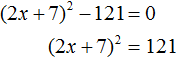
Let's use the square root:
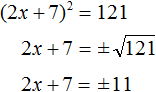
We get two simple equations: 2x + 7 = 11 and 2x + 7 = -11. Solve them:
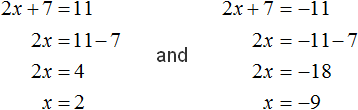
Example 5. Solve the equation 2x2 + 3x - 27 = 0
This equation is a little more complicated. When we extract the full square, we represent the first term of the quadratic trinomial as the square of some expression.
So, in the last example, the first term of the equation was 4x2. It could be represented as the square of the expression 2x, that is, (2x)2 = 22x2 = 4x2. To see if this is correct, you can take the square root of the expression 4x2. This is the square root of the product - it is equal to the product of the roots:
In the equation 2x2 + 3x - 27 = 0, the first term is 2x2. It cannot be represented as the square of any expression. Because there is no number whose square is 2. If there were such a number, it would be the square root of 2. But the square root of number 2 can only be approximated. And an approximation is not a good way to represent number 2 as a square.
If both parts of the original equation are multiplied or divided by the same number, you get an equation that is equal to the original equation. This rule holds for the quadratic equation as well.
Then we can divide both parts of our equation by 2. This will get rid of the two in front of x2, which will then allow us to isolate the complete square:
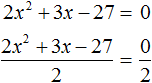
Rewrite the left part as three fractions with a denominator of 2
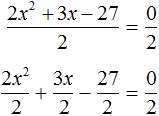
Reduce the first fraction by 2. The remaining terms of the left part will be rewritten without change. The right part will still be equal to zero:
Let's select a complete square.
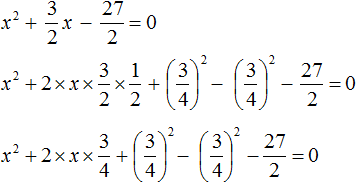
If the term 
were presented as a doubled product, the appearance of factor 2 would cause that factor and the denominator of fraction 
to be reduced. To prevent this from happening, the doubled product was multiplied by 
. When you extract a full square, you should always try to make sure that the value of the original expression does not change.
Let's fold the resulting complete square:
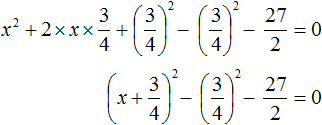
Here are some like terms:
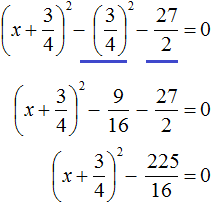
Move the fraction 
to the right side, changing the sign:
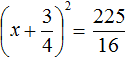
Let's use the square root. Expression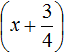 
is the square root of 
To calculate the right part, use the rule of extraction of the square root of the fraction:
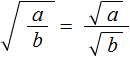
Then our equation will look like this:
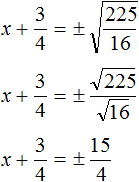
We get two equations:
Let's solve them:
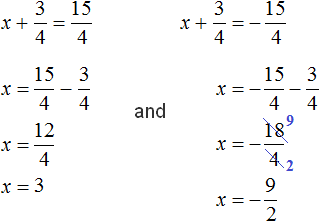
So the roots of the equation 2x2 + 3x - 27 = 0 are the numbers 3 and 
.
It is more convenient to leave the root in this form without dividing the numerator by the denominator. This makes it easier to check.
Let's check it. Substitute the found roots in the original equation:
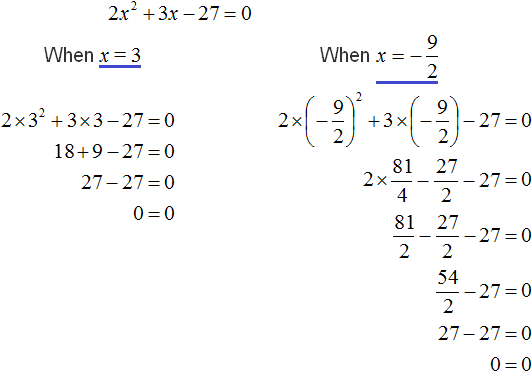
In both cases the left-hand side is zero, so the equation 2x2 + 3x - 27 = 0 is solved correctly.
Solving the equation 2x2 + 3x - 27 = 0, at the very beginning we divided both parts of it by 2. The result is a quadratic equation in which the coefficient in front of x2 is equal to one:
This kind of quadratic equation is called a reduced quadratic equation.
Any quadratic equation of the form ax2 + bx + c = 0 can be made reduced. To do this, divide both parts of it by the coefficient in front of x². In this case, both parts of the equation ax2 + bx + c = 0 must be divided by a
Example 6. Solve the quadratic equation 2x2 + x + 2 = 0
Let us make this equation reduced:
Let's select a complete square:
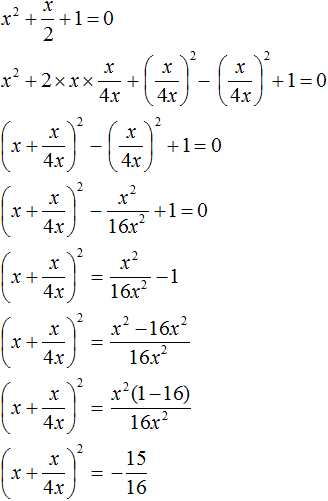
We obtained equation 
, in which the square of expression 
is equal to the negative number 
. This cannot be the case since the square of any number or expression is always positive.
Consequently, there is no value of x at which the left-hand side equals . So equation has no roots.
And since equation is equivalent to the original equation 2x2 + x + 2 = 0, it (the original equation) has no roots either.
Root formulas for a quadratic equation
It is not very convenient to allocate a complete square for each quadratic equation to be solved.
Is it possible to create universal formulas for solving quadratic equations? It turns out you can. That's what we're going to do now.
By taking the letter equation ax2 + bx + c = 0, and performing some identical transformations, we can obtain formulas for deriving the roots of the quadratic equation ax2 + bx + c = 0. We can substitute the coefficients a, b, c into these formulas and get ready solutions.
So let us extract the full square from the left part of the equation ax2 + bx + c = 0. First we make this equation reduced. Divide both parts of it by a
Now in the resulting equation let's select the full square:
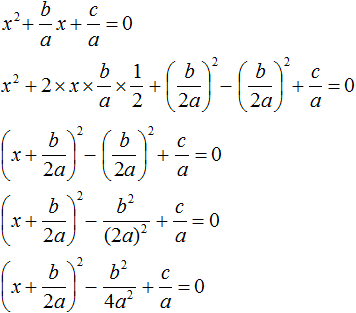
Move terms 
and 
to the right side, changing the sign:
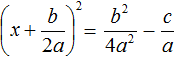
Let's reduce the right part to a common denominator. Fractions consisting of letters are reduced to a common denominator using the "crosswise" method. That is, the denominator of the first fraction becomes an additional factor of the second fraction, and the denominator of the second fraction becomes an additional factor of the first fraction:
In the numerator of the right-hand side, bracket a
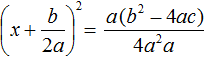
Let's reduce the right part by a
Since all transformations were identical, the resulting equation 
has the same roots as the original equation ax2 + bx + c = 0.
Equation will have roots only if the right-hand side is greater than or equal to zero. This is because the left part is squared, and the square of any number is positive or equal to zero (if zero is squared). What the right-hand side equals depends on what is substituted for variables a, b and c.
Since for any a not equal to zero, the denominator of the right-hand side of equation will always be positive, the sign of fraction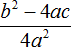 
will depend on the sign of its numerator, that is, on the expression b2 − 4ac.
The expression b2 − 4ac is called the discriminant of a quadratic equation. The discriminant is a Latin word. The discriminant of a quadratic equation is denoted by the letter D
D = b2 − 4ac
The discriminant allows you to know in advance whether an equation has roots or not. For example, in the previous problem we solved the equation 2x2 + x + 2 = 0 and it turned out to have no roots. The discriminant, on the other hand, would have let us know in advance that there were no roots. In the equation 2x2 + x + 2 = 0, the coefficients a, b, and c are 2, 1, and 2, respectively. Let us substitute them into the formula D = b2−4ac
D = b2 − 4ac = 12 − 4 × 2 × 2 = 1 − 16 = −15.
We see that D (aka b2 − 4ac) is a negative number. Then there is no point in solving the equation 2x2 + x + 2 = 0, allocating the full square in it, because when we get to the equation of the form , it turns out that the right side is less than zero (because of the negative discriminant). And the square of a number cannot be negative. Consequently, this equation will have no roots.
It becomes clear why ancient people considered the expression b2 − 4ac a distinguisher. This expression is like an indicator to distinguish between an equation that has roots and an equation that has no roots.
So, D is equal to b2 − 4ac. Substitute the letter D in equation instead of the expression b2 − 4ac
If the discriminant of the original equation is less than zero (D < 0), the equation will take the form:
In this case the original equation is said to have no roots since the square of any number must not be negative.
If the discriminant of the original equation is greater than zero (D > 0), the equation will take the form:
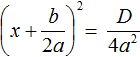
In this case the equation will have two roots. We will use the square root to derive them:
You have the equation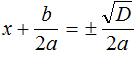 
. From it we obtain two equations: 
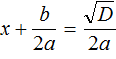and 
. Express x in each of the equations:
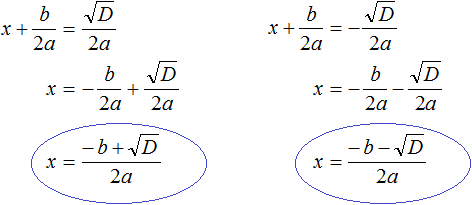
The resulting two equations are universal formulas for solving the quadratic equation ax2 + bx + c = 0. They are called formulas for the roots of a quadratic equation.
Most often these formulas are denoted as x1 and x2. That is, the formula with index 1 is used to calculate the first root; the formula with index 2 is used to derive the second root. Let us denote our formulas in the same way:
The order in which the formulas are applied is not important.
Solve for example the quadratic equation x2 + 2x − 8 = 0 using the formulas for the roots of the quadratic equation. The coefficients of this quadratic equation are the numbers 1, 2, and -8. That is, a = 1, b = 2, c = -8.
Before you can use the formulas for the roots of a quadratic equation, you must find the discriminant of the equation.
Find the discriminant of the quadratic equation. To do this, we use the formula D = b2 − 4ac. Instead of variables a, b and c we will have the coefficients of the equation x2 + 2x − 8 = 0
D = b2 − 4ac = 22− 4 × 1 × (−8) = 4 + 32 = 36
The discriminant is greater than zero. So the equation has two roots. Now you can use the formulas for the roots of a quadratic equation:
So the roots of the equation x2 + 2x − 8 = 0 are 2 and -4. Check to make sure that the roots are found correctly:
Finally, consider the case where the discriminant of the quadratic equation is zero. Let us return to the equation . If the discriminant is zero, then the right-hand side of the equation will look like this:
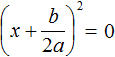
And in this case the quadratic equation will have only one root. Let's use the square root:
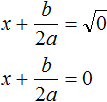
Then we express x
This is another formula for deriving the square root. Let's look at its application. Earlier we solved the equation x2 − 6x + 9 = 0, which has one root of 3. We solved it using the method of extraction of the full square. Now let's try to solve it using formulas.
Find the discriminant of the quadratic equation. In this equation a = 1, b = -6, c = 9. Then by the discriminant formula we have:
D = b2 − 4ac = (−6)2 − 4 × 1 × 9 = 36 − 36 = 0
The discriminant is zero (D = 0). This means that the equation has only one root, and it is calculated using the formula 
So the root of the equation x2 − 6x + 9 = 0 is number 3.
For a quadratic equation that has one root, the formulas 
and 
also apply. But the application of each of them will give the same result.
Let us apply these two formulas to the previous equation. In both cases we get the same answer 3
If a quadratic equation has only one root, it is preferable to use the formula rather than the and formulas. This saves time and space.
Example 3. Solve the equation 5x2 - 6x + 1 = 0
Find the discriminant of the quadratic equation:
The discriminant is greater than zero. So the equation has two roots. Use the formulas for the roots of a quadratic equation:
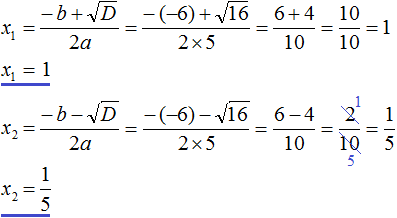
So the roots of the equation 5x2 - 6x + 1 = 0 are numbers 1 and 
.
Answer: 1; .
Example 4. Solve the equation x2 + 4x + 4 = 0
Find the discriminant of the quadratic equation:
The discriminant is zero. So the equation has only one root. It is calculated by the formula
So the root of the equation x2 + 4x + 4 = 0 is -2.
Answer: -2.
Example 5. Solve the equation 3x2 + 2x + 4 = 0
Find the discriminant of the quadratic equation:
The discriminant is less than zero. So this equation has no roots.
Answer: no roots.
Example 6. Solve the equation (x + 4)2 = 3x + 40
Let us reduce this equation to the normal form. On the left side is the square of the sum of the two expressions. Let's expand it:
Move all the terms from the right part to the left part by changing their signs. The right side will contain zero:
Let's give like terms on the left side:
In the resulting equation, find the discriminant:
The discriminant is greater than zero. So the equation has two roots. Use the formulas for the roots of a quadratic equation:
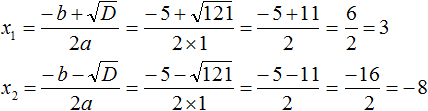
So the roots of the equation (x + 4)2 = 3x + 40 are the numbers 3 and -8.
Answer: 3; −8.
Example 7. Solve the equation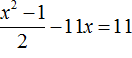 
Let's multiply both parts of this equation by 2. This will allow us to get rid of the fraction on the left side:
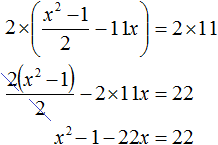
In the resulting equation, move 22 from the right side to the left side, changing the sign. The right side will remain 0
Let's give like terms on the left side:
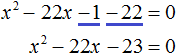
In the resulting equation, find the discriminant:
The discriminant is greater than zero. So the equation has two roots. Use the formulas for the roots of a quadratic equation:
So the roots of equation 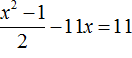are the numbers 23 and -1.
Answer: 23; −1.
Example 8. Solve equation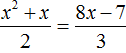 
Multiply both parts by the least common multiple of the denominators of both fractions. This will get rid of the fractions in both parts. The least common multiple of 2 and 3 is 6:
In the resulting equation, open the brackets in both parts:
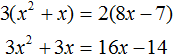
Now move all the terms from the right side to the left side by changing their signs. The right hand side will contain 0
Let's give similar terms on the left side:
In the resulting equation, find the discriminant:
The discriminant is greater than zero. So the equation has two roots. Use the formulas for the roots of a quadratic equation:
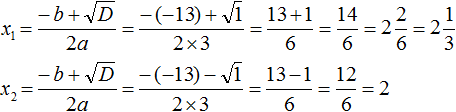
So the roots of equation 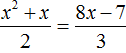are the numbers 
and 2.
Examples of solving quadratic equations
Example 1. Solve the equation x2 = 81
This is a simple quadratic equation in which you have to determine the number whose square is 81. These are numbers 9 and -9. Use the square root to derive them:
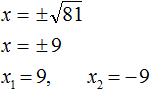
Answer: 9, −9.
Example 2. Solve the equation x2 − 9 = 0
This is an incomplete quadratic equation. To solve it, move the term -9 to the right side, changing the sign. Then we get:
Answer: 3, −3.
Example 3. Solve the equation x2 − 9x = 0
This is an incomplete quadratic equation. To solve it, move the term -9 to the right side, changing the sign. Then we get:
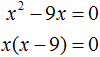
The left side of the equation is a product. A product is zero if at least one of the factors is zero.
The left part will become zero if x is zero separately, or if the expression x - 9 is zero. We get two equations, one of which is already solved:
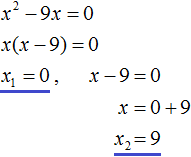
Answer: 0, 9.
Example 4. Solve the equation x2 + 4x − 5 = 0
This is a complete quadratic equation. It can be solved by the full square extraction method or by using the formulas for the roots of the quadratic equation.
Let's solve this equation using formulas. First we find the discriminant:
D = b2 − 4ac = 42 − 4 × 1 × (−5) = 16 + 20 = 36
The discriminant is greater than zero. So the equation has two roots. Let's calculate them:
Answer: 1, −5.
Example 5. Solve the equation 
Multiply both parts by the least common multiple of 5, 3, and 6. This will get rid of the fractions in both parts:
In the resulting equation, move all the terms from the right side to the left side, changing the sign. The right side will remain zero:
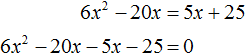
Here are some like terms:
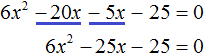
Solve the resulting equation using formulas:
Answer: 5, 
.
Example 6. Solve the equation x2 = 6
In this example, as in the first one, you need to use the square root:
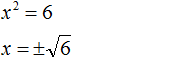
However, the square root of number 6 is not extracted. It is only extracted approximately. The root can be extracted with a certain accuracy. Extract it to the nearest hundredth:
But more often than not, the root is left as a radical:
Answer: 
Example 7. Solve the equation (2x + 3)2 + (x − 2)2 = 13
Open the brackets on the left side of the equation:
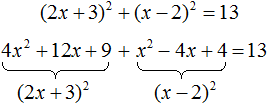
In the resulting equation, move 13 from the right side to the left side, changing the sign. Then we give like terms:
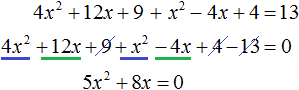
We got an incomplete quadratic equation. Let's solve it:
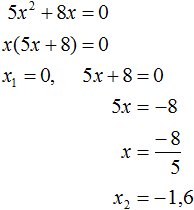
Answer: 0, −1,6.
Example 8. Solve the equation (5 + 7x)(4 − 3x) = 0
This equation can be solved in two ways. Let us consider each of them.
First way. Open the brackets and obtain the normal form of the quadratic equation.
Let's open the brackets:
Let's give some like terms:
Let us rewrite the resulting equation so that the term with the higher coefficient is placed first, the term with the second coefficient is placed second, and the free term is placed third:
To make the leading term positive, multiply both parts of the equation by -1. Then all terms of the equation will change their signs to the opposite:
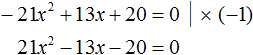
Solve the resulting equation using the formulas for the roots of the quadratic equation:
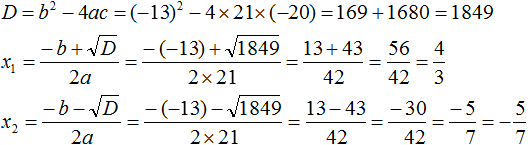
The second way. Find the values of x, at which the factors of the left part of the equation are equal to zero. This way is more convenient and much shorter.
A product is zero if at least one of the factors is zero. In this case, the equality in the equation (5 + 7x)(4 - 3x) = 0 will be achieved if the expression (5 + 7x) is zero, or if the expression (4 - 3x) is zero. Our task is to find out at which x this happens:
Examples of task solutions
Let's imagine that it is necessary to build a small room with an area of 8 m2. The length of the room must be twice its width. How to determine the length and width of such a room?
Let's make a rough drawing of this room, which illustrates the view from above:
Denote the width of the room by x. And the length of the room by 2x, because by the condition of the problem the length must be twice as big as the width. The multiplier 2 will satisfy this requirement:
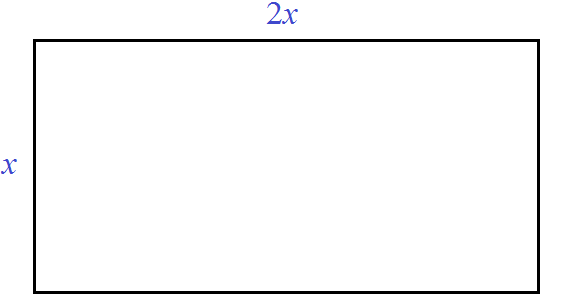
The surface of the room (its floor) is a rectangle. To calculate the area of a rectangle, multiply the length of the rectangle by its width. Let's do this:
2x × x
According to the problem, the area must be 8 m2. So the expression 2x × x must be equated with 8
2x × x = 8
You get an equation. If you solve it, you can find the length and width of the room.
The first thing you can do is perform multiplication on the left side of the equation:
2x2 = 8
As a result of this transformation, the variable x has been converted to the second power. And we said that if a variable in an equation is raised to the second power (squared), then such an equation is a second-power equation or a quadratic equation.
To solve our quadratic equation, we will use the identity transformations we studied earlier. In this case we can divide both parts by 2
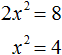
Now let us use the square root. If x2 = 4, then . Hence x = 2 and x = -2.
x is the width of the room. The width must not be negative, so we only take the value of 2. This often happens when solving problems in which a quadratic equation is applied. The answer yields two roots, but only one of them satisfies the problem.
And the length was denoted by 2x. The value of x is now known, let's substitute it into the expression 2x and calculate the length:
2x = 2 × 2 = 4
So the length is 4 m and the width is 2 m. This solution satisfies the condition of the task, because the area of the room is 8 m2
4 × 2 = 8 m2
Answer: The length of the room is 4 m and the width is 2 m.
Example 2. A rectangular garden plot, one side of which is 10 m larger than the other, needs a fence. Determine the length of the fence if you know that the area of the plot is 1200 m2
Solution
The length of a rectangle is usually greater than its width. Let the width of the plot be x meters, and the length (x + 10) meters. The area of the plot is 1200 m2. Multiply the length of the plot by its width and equate it to 1200, you get the equation:
x(x + 10) = 1200
Let us solve this equation. First, let's solve the brackets in the left part of the equation:
Move 1200 from the right side to the left side, changing the sign. The right side will remain 0
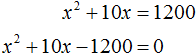
Solve the resulting equation using formulas:
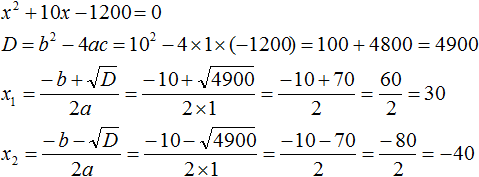
Even though the quadratic equation has two roots, we only take the value 30. Because the width cannot be expressed by a negative number.
So, x is the width of the plot. It is equal to thirty meters. And the length was denoted by the expression x + 10. Let's substitute the found value of x into it and calculate the length:
x + 10 = 30 + 10 = 40 m
So the length of the plot is forty meters and the width is thirty meters. These values satisfy the condition of the problem, because if you multiply the length and width (numbers 40 and 30) you get 1200 m2
40 × 30 = 1200 m2
Now answer the question of the problem. What is the length of the fence? In order to calculate it, you need to find the perimeter of the plot.
The perimeter of a rectangle is the sum of all its sides. Then:
P = 2(a + b) = 2 × (40 + 30) = 2 × 70 = 140 m.
Answer: The length of the hedge of the garden plot is 140 m.
.
2. If you find an error or inaccuracy, please describe it.
3. Positive feedback is welcome.